一、报告题目：Schauder fixed point theorem and Krein–Milman theorem in random normed modules and their applications
二、报告人：郭铁信教授
三、报告时间：2022年11月22日（星期二）16：00-18：00
四、报告平台：腾讯会议（会议ID：459869178）
五、报告摘要: Motivated by the randomized version of the classical Bolzano–Weierstrass theorem, in this paper we first introduce the notion of a random sequentially compact set in a general random normed module (briefly, an RN module, which is a random generalization of an ordinary normed space) and systematically develop the related theory. For an RN module E and a random sequentially compact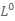 
–convex subset G of E, we then establish the Schauder fixed point theorem: every σ–stable continuous mapping f of G to G has a fixed point, and the Krein–Milman theorem: G is the closed 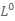–convex hull of the set of 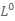–extreme points of G. Finally, as an application of the two theorems, we prove the existence of Nash equilibrium points in the context of conditioning information. It should be pointed out that random sequentially compact sets are very often noncompact and thus our whole paper is almost centered on the difficulty in overcoming noncompactness.
六、报告人简介：郭铁信，男，出生于1965年2月13日。1982年至1992年在西安交通大学数学系获理学学士、硕士与博士学位;1992年至1994年在四川大学数学系作博士后研究工作;在1994年至2006年在厦门大学数学科学学院任教授与博士生导师;期间2001年至2002年为麻省理工学院数学系访问教授; 2006年至2012年在北京航空航天大学数学与系统科学学院任二级教授，2012年作为高层次引进人才到中南大学工作，现为中南大学数学与统计学院二级教授与科研副院长、中国工业与应用数学学会金融数学与金融工程专业委员会委员、湖南省数学会常务理事。长期从事Banach空间理论、随机泛函分析及其在金融数学中应用的研究，原创性的提出了随机赋范模等基本框架并奠定了该理论随后发展的基础，这些工作被西方金融学者称为开创性工作。近年来一直致力于开拓随机泛函分析在Banach空间理论、随机微分方程与金融风险理论中的应用。主 持国家自然科学基金面上项目6项，在国内外专业领域的著名杂志《J.Funct.Anal.》、《Sci.China Math.》、《J.Geom.Anal.》、《J.Appr.Theory》、《J.Math.Anal.Appl.》与《Nonlinear Anal.》等上发表论文40多篇。单独获福建省科技进步二等奖与香港运盛青年科技奖。应邀在第八届世界华人数学家大会与中国数学会年会上作45分钟邀请报告。
